L’addition, la soustraction, la multiplication et la division décimale et euclidienne à travers une série d’exercices de maths en 6ème corrigés sur les 4 opérations. L’élève devrai maîtriser les quatre opération et savoir poser en ligne ou colonne une addition, une soustraction, une multiplication ou une division euclidienne et décimale. Il devra maitriser, également, les différents critères de divisibilité ainsi que le vocabulaire de la somme, de la différence, du produit et du quotient. En 6ème, les élèves apprennent à utiliser les quatre opérations fondamentales de l’arithmétique : l’addition, la soustraction, la multiplication et la division.
Exercice 1
Calculer mentalement :
a. 0,065×10 b. 79,2x 1 000 c. 71,47 x 100 d. 0,34 x 1 000 e. 87 x 100
f. 934 x 10 g. 0,001 x 1 000 h. 0,05 x 10 000.
Exercice 2
Convertir les longueurs suivantes :
a. 84 km en m. b. 84 hm en m. c. 84 mm en m. d. 84 dm en m.
e. 3,1 hm en km. f. 3,1 dam en m. g. 3,1 m en hm.
Exercice 3
Calculer mentalement les additions suivantes :
a. 5,6 + 7,2 b. 2,2 + 6,3 c. 3,5 + 7,1 d. 6,3 + 7,6 e. 9+ 3,5 f. 2,9 + 6,5 g. 5,8 + 9,3
Exercice 4
Calculer mentalement les soustractions suivantes :
a. 5,5 – 4,3 b. 4,6 – 0,5 c. 2,8 – 2,1 d. 6,7 – 0,5 e. 3,8 – 3,5 f. 4,6 – 2,8 g. 9,7 – 5,9.
Exercice 5
Placer la virgule dans le nombre écrit en caractère gras pour que l’égalité soit vraie.
Exercice 6
Poser les multiplications suivantes et calculer.
Exercice 7
Recopier puis placer correctement la virgule en rouge dans le nombres souligné :
1/ 75,315×4,83=36377145
2/ 1,08×471591=509,31838
3/ 54026×247=133,44422
4/ 0,861597×552,5=4760323425
Exercice 8
Poser la multiplication pour calculer :
• le produit de 5,4 par 60,9 ;
• le produit de 7,536 par 8,6 .
Exercice 9
Recopier puis donner le résultat :
1/ 45×10=…
2/ 57,6×0,1=…
3/ 5,69×0,001=…
4/ 8,67×1000=…
5/ 12,1×10=…
6/ 0,089×100=…
7/ 0,089×100=…
8/ 100×0,0001=…
Exercice 10
Calculer les produits suivants en faisant des regroupements astucieux :
1/ A=4×3,98×25×10
2/ B=8×7,1234×25
3/ Donner la propriété fondamentale du cours qui vous a permis d’effectuer ces calculs.
Exercice 11
Effectuer les divisions décimales suivantes afin d’en trouver le quotient décimal exact.
Exercice 12
1/ Pour un concert, dix-mille billets ont été vendus.
Le billet coûte 11 € 20. Quelle est la recette ?
2/ Un train de dix wagons transporte des voitures dont le prix à l’unité est de 0,014850 millions d’euros.
Il y a dix voitures par wagon.
Quelle somme d’argent représente l’ensemble des voitures contenues dans ce train ?
Exercice 13
Calculer en regroupant astucieusement les termes.
a. 25+ 128+175+22
b. 357 + 149 + 43 +51
c. 2,45 + + 1,55 + 14,2
d. 7,48 + 1,03 + 4,52 + 3,47
Exercice 14
Poser l’opération, puis vérifier à la calculatrice.
a.
b.
c.
d.
Exercice 15
Poser les différentes soustractions en colonne puis; vérifier les résultats
à l’aide de votre calculatrice.
a.
b.
c.
d.
Exercice 16
Voici la photo d’un iceberg dont la hauteur totale est estimée à 267 m.
La hauteur apparente hors d’eau est de 29,83 m.
a. Donner un ordre de grandeur de la hauteur de la partie immergée.
b. Calculer la valeur exacte de cette hauteur.
Exercice 17
Poser ces différentes multiplications en colonne, puis, vérifier les résultats à l’aide de la calculatrice.
a.
b.
c.
d.
e.
f.
g.
h.
Exercice 18
Voici les prix au kilogramme de quelques fruits et la quantité achetée.
Pour chaque fruit, calculer le prix à payer.
Exercice 19
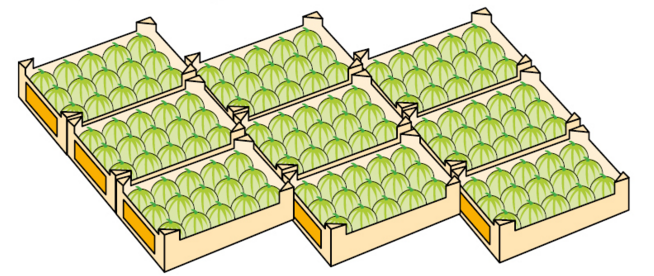
Voici les caisses de melons présentes sur un étalage.
Chaque caisse vide pèse 0,7 kg et chaque melon pèse en moyenne 600 g.
a. Écrire une expression qui permet de calculer la masse totale, en kg, de ces caisses.
b. Calculer cette masse totale.
c. Les expressions obtenues dans la classe sont-elles toutes les mêmes ?
Exercice 20
Carine, Lilou, Laurie, Marc et Adrien ont acheté des mâts pour leurs voiliers.
Le mât du voilier de Carine mesure 2,4 m.
Celui du voilier de Lilou est deux fois et demie plus long que celui du voilier de Carine.
Celui du voilier de Laurie mesure 1 m de moins que celui du voilier de Lilou.
Celui du voilier de Marc mesure un mètre et demi de plus que celui du voilier de Laurie.
Celui du voilier de Lilou est une fois et demie moins long que celui du voilier d’Adrien.
Calculer la longueur de chaque mât.
Télécharger puis imprimer cette fiche en PDF.
Télécharger ou imprimer cette fiche «addition, soustraction, multiplication et division : exercices en de maths 6ème corrigés en PDF.» au format PDF afin de pouvoir travailler en totale autonomie.
D'autres cours et exercices à consulter
- Triangles et quadrilatères : exercices de maths en 6ème corrigés en PDF.
- Nombres décimaux : exercices de maths en 6ème corrigés en PDF.
- L’aire et le périmètre : exercices de maths en 6ème corrigés en PDF.
- Droites parallèles et perpendiculaires : exercices de maths en 6ème corrigés en PDF.
- Cercle : exercices de maths en 6ème corrigés en PDF.
- Fractions : exercices de maths en 6ème corrigés en PDF.
- Proportionnalité : exercices en 6ème de maths corrigés en PDF.
- Bissectrice d’un angle : exercices de maths en 6ème corrigés en PDF.